Calculus Exercise (8) : Derivative of Trigonometric Functions
Trigonometric Limits
- $\displaystyle \lim_{x \to 0} \left( {\sin x} \right)=0$
- $\displaystyle \lim_{x \to 0} \left(\cos x\right)=1$
- $\displaystyle \lim_{x \to 0} \left(\dfrac{\sin x}{x}\right)=1$
ဖော်ပြပါပုံသေနည်း များဖြစ်ပေါ်လာပုံကို ဒီနေရာမှာ ဖတ်ပါ။
Derivative of $\sin x$ and $\cos x$ from first principles
အထက်ဖော်ပြပါ limit များကို အသုံးချ၍ $\sin x$ နှင့် $\cos x$ ၏ derivatives များကို အောက်ပါအတိုင်း ရှာယူနိုင်ပါသည်။
$$\begin{aligned} y &=\sin x \\\\ y+\delta y &=\sin (x+\delta x) \\\\ \delta y &=(y+\delta y)-y \\\\ &=\sin (x+\delta x)-\sin x \\\\ &=2 \cos \frac{x+\delta x+x}{2} \sin \frac{x+\delta x-x}{2} \\\\ \frac{\delta y}{\delta x} &=\frac{2}{\delta x} \cos \left(x+\frac{\delta x}{2}\right) \sin \frac{\delta x}{2} \\\\ &=\cos \left(x+\frac{\delta x}{2}\right) \frac{\sin \frac{\delta x}{2}}{\frac{\delta x}{2}} \\\\ \frac{d y}{d x} &=\lim _{\delta x \rightarrow 0} \frac{\delta y}{\delta x} \\\\ &=\lim _{\delta x \rightarrow 0}\left[\cos \left(x+\frac{\delta x}{2}\right) \cdot \frac{\sin \frac{\delta x}{2}}{\frac{\delta x}{2}}\right] \\\\ &=\cos (x+0)(1) \\\\ &=\cos x \end{aligned}$$ $$\begin{array}{|c|} \hline \dfrac{d}{d x}(\sin x)=\cos x\\ \hline \end{array}$$ $$\begin{aligned} y &=\cos x \\\\ y+\delta y &=\cos (x+\delta x) \\\\ \delta y &=(y+\delta y)-y \\\\ &=\cos (x+\delta x)-\cos x \\\\ &=-2 \sin \left(\frac{x+\delta x+x}{2}\right) \sin \left(\frac{x+\delta x-x}{2}\right) \\\\ \frac{\delta y}{\delta x} &=-\frac{2}{\delta x} \sin \left(x+\frac{\delta x}{2}\right) \sin \frac{\delta x}{2} \\\\ &=-\sin \left(x+\frac{\delta x}{2}\right) \frac{\sin \frac{\delta x}{2}}{\frac{\delta x}{2}} \\\\ \frac{d y}{d x} &=\lim _{\delta x \rightarrow 0} \frac{\delta y}{\delta x} \\\\ &=\lim _{\delta x \rightarrow 0}\left[-\sin \left(x+\frac{\delta x}{2}\right) \cdot \frac{\sin \frac{\delta x}{2}}{\frac{\delta x}{2}}\right] \\\\ &=-\sin x \end{aligned}$$ $$\begin{array}{|c|} \hline \dfrac{d}{d x}(\cos x)=-\sin x\\ \hline \end{array}$$ |
Drivative of Other Trigonometric Functions
$\sin x$ နှင့် $\cos x$ ၏ derivatives များကို သိလျှင် အခြား trigonometric fuctions များ ဖြစ်ကြသော
$\tan x, \cot x, \sec x$ နှင့် $\csc x$ တို့၏ derivatives များကို chain rule, product rule, quotient
rule များကို သုံး၍ အလွယ်တကူရှာနိုင်ပါသည်။
Derivative of $\tan x$$$ \begin{aligned} \frac{d}{d x}(\tan x) &=\frac{d}{d x}\left(\frac{\sin x}{\cos x}\right) \\\\ &=\frac{\cos x \frac{d}{d x}(\sin x)-\sin x \frac{d}{d x}(\cos x)}{\cos ^{2} x} \\\\ &=\frac{\cos ^{2} x+\sin ^{2} x}{\cos ^{2} x} \\\\ &=\frac{1}{\cos ^{2} x} \\\\ &=\sec ^{2} x \end{aligned} $$ Derivative of $\cot x$$$\begin{aligned} \dfrac{d}{d x}(\cot x) &=\dfrac{d}{d x}\left(\dfrac{\cos x}{\sin x}\right) \\\\ &=\dfrac{\sin x \dfrac{d}{d x}(\cos x)-\cos x \dfrac{d}{d x}(\sin x)}{\sin ^{2} x} \\\\ &=\dfrac{-\sin ^{2} x-\cos ^{2} x}{\sin ^{2} x} \\\\ &=\dfrac{-\left(\sin ^{2} x+\cos ^{2} x\right)}{\sin ^{2} x} \\\\ &=-\dfrac{1}{\sin ^{2} x} \\\\ &=-\csc^{2} x \end{aligned}$$ Derivative of $\sec x$$$\begin{aligned} \frac{d}{d x}(\sec x) &=\frac{d}{d x}\left(\frac{1}{\cos x}\right) \\\\ &=-\frac{1}{\cos ^{2} x} \frac{d}{d x}(\cos x) \\\\ &=-\frac{1}{\cos ^{2} x}(-\sin x) \\\\ &=\frac{1}{\cos x} \cdot \frac{\sin x}{\cos x} \\\\ &=\sec x \cdot \tan x \end{aligned}$$ Derivative of $\csc x$$$\begin{aligned} \frac{d}{d x}(\csc x) &=\frac{d}{d x}\left(\frac{1}{\sin x}\right) \\\\ &=-\frac{1}{\sin ^{2} x} \frac{d}{d x}(\sin x) \\\\ &=-\frac{1}{\sin x} \frac{\cos x}{\sin x} \\\\ &=-\csc x \cot x \end{aligned}$$ |
Formulas for Derivatives of Trigonometric Functions
$$\begin{array}{|l|l|l|} \hline 1 & \dfrac{d}{d x} \sin x=\cos x & \dfrac{d}{d x} \sin u=\cos u \dfrac{d u}{d x} \\ \hline 2 & \dfrac{d}{d x} \cos x=-\sin x & \dfrac{d}{d x} \cos u=-\sin u \dfrac{d u}{d x} \\ \hline 3 & \dfrac{d}{d x} \tan x=\sec ^{2} x & \dfrac{d}{d x} \tan u=\sec ^{2} u \dfrac{d u}{d x} \\ \hline 4 & \dfrac{d}{d x} \cot x=-\csc^{2} x & \dfrac{d}{d x} \cot u=-\csc^{2} u \dfrac{d u}{d x} \\ \hline 5 & \dfrac{d}{d x} \sec x=\sec x \tan x & \dfrac{d}{d x} \sec u=\sec u \tan u \dfrac{d u}{d x} \\ \hline 6 & \dfrac{d}{d x} \csc x=-\csc x \cot x & \dfrac{d}{d x} \csc u=-\csc u \cot u \dfrac{d u}{d x} \\ \hline \end{array}$$ |
Problems
Differentiate the following with respect to $x$.
$\begin{aligned}
&\\
\text {(a) }\quad & \sin 5 x\\\\
\text {(b) }\quad & \cos \left(7 x^{2}-2\right)\\\\
\text {(c) }\quad & \tan (6 x+7)\\\\
\text {(d) }\quad & 5 \sec (3 x+1)\\\\
\text {(e) }\quad & \sin (2 x+3) \\\\
\text {(f) }\quad & \cos \left(\dfrac{3}{x}\right)\\\\
\text {(g) }\quad & x^{3} \cos 2 x\\\\
\text {(h) }\quad & \cos 7 x+\sin 3 x\\\\
\text {(i) }\quad & \dfrac{\cot (1-2 x)}{3}\\\\
\text {(j) }\quad & -2 \csc 3 x\\\\
\text {(k) }\quad & \sin x \cos 2 x\\\\
\text {(l) }\quad & \cos ^{2}(5 x)\\\\
\text {(m) }\quad & \tan ^{3} \sqrt{x}\\\\
\text {(n) }\quad & \sin (\cos x)\\\\
\text {(o) }\quad & \dfrac{\sin x}{1+\tan x}\\\\
\text {(p) }\quad & \sqrt{\sin x+\cos x}\\\\
\text {(q) }\quad & (x+\tan x)^{3}\\\\
\text {(r) }\quad & \dfrac{\tan 2 x}{1+\cot x}
\end{aligned}$
Find $\dfrac{d y}{d x}$.
$\begin{aligned}
&\\
\text {(a) }\quad & y=\sin^2x\\\\
\text {(b) }\quad & y=\cos \sqrt{x} \\\\
\text {(c) }\quad & y=\tan ^{2}\left(x^{2}\right) \\\\
\text {(d) }\quad & y=\sin 2x – x \cos x\\\\
\text {(e) }\quad & y=\dfrac{x}{\tan x}\\\\
\text {(f) }\quad & y=\sin x \cos ^{2} x\\\\
\text {(g) }\quad & y=\sin \left(1-x^{2}\right)\\\\
\text {(h) }\quad & y=2 \pi x+2 \cos \pi x\\\\
\text {(i) }\quad & y=\sin ^{2} x \cos {3 x}\\\\
\text {(j) }\quad & y=x^{2} \sin \left(\dfrac{1}{x}\right)\\\\
\text {(k) }\quad & 3 x^{2}+2 \sin y=y^{2}\\\\
\text {(l) }\quad & \sin x \cos y=2 y\\\\
\text {(m) }\quad & 2 x y+\sin x=3\\\\
\text {(n) }\quad & y=\dfrac{\cos (1-2 x)}{\sqrt{x}}\\\\
\text {(o) }\quad & y=\sin ^{4} x \cos (3 x)\\\\
\text {(p) }\quad & x+\sin y=\cos (x y)\\\\
\text {(q) }\quad & x+y^{2}=\sin (x+y)\\\\
\text {(r) }\quad & 2 \sin x=x+\cos y
\end{aligned}$
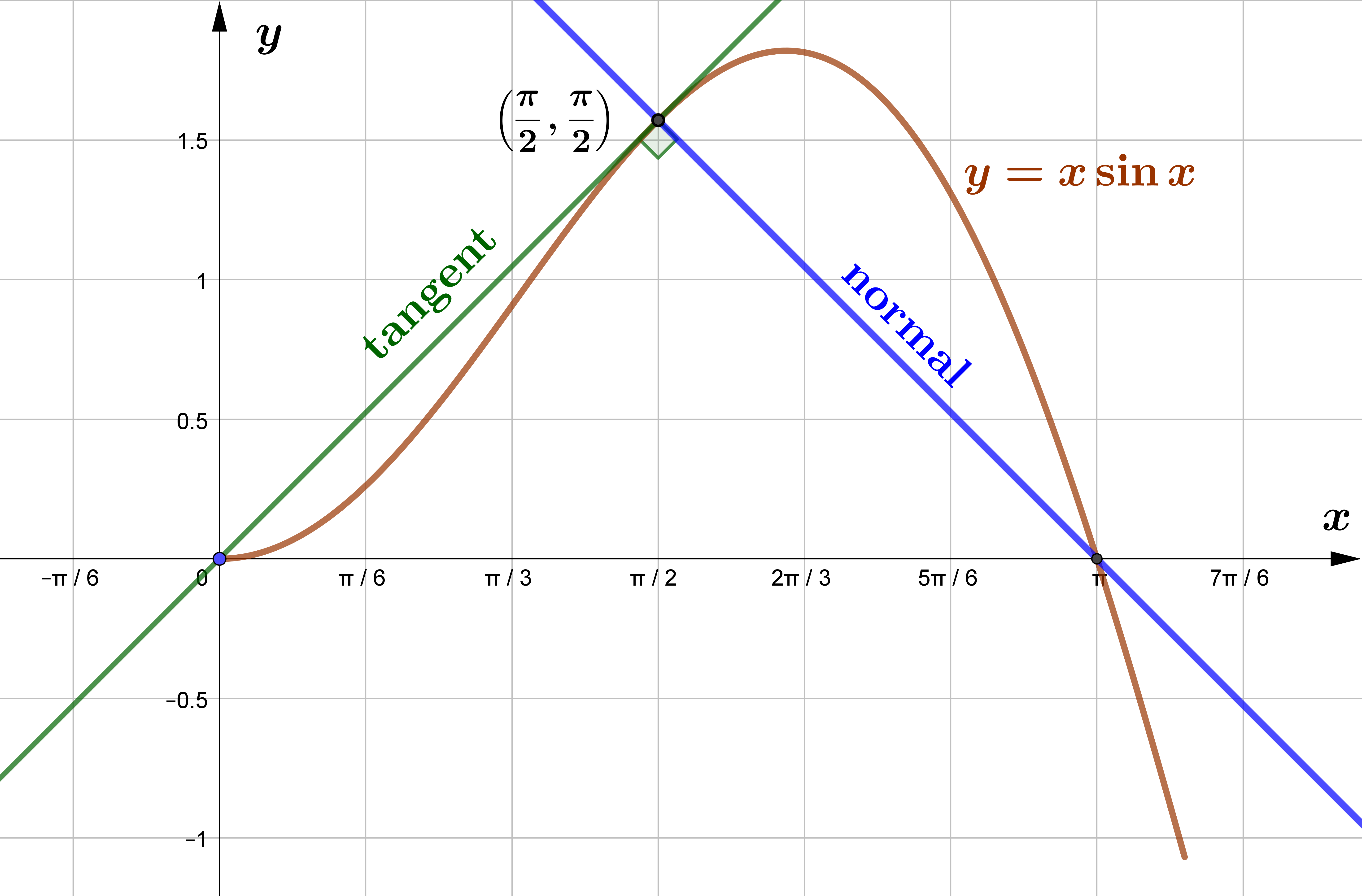
Given that $y=x \sin x$, find $\dfrac{d^{2} y}{d x^{2}}$.
Given that $y=\cos ^{2} x$, prove that $\dfrac{d^{2} y}{d x^{2}}+4 y=2$.
Given that $y=\dfrac{1}{3} \cos ^{3} x-\cos x$, prove that $\dfrac{d y}{d x}=\sin ^{3} x$.
If $x \cos y=\sin x$, prove that $\dfrac{d y}{d x}=\dfrac{\cos y(\cos y-\cos x)}{\sin y \sin x}$.
SOLUTION
$\begin{aligned}
\text{(a)}\quad & \dfrac{d}{d x}(\sin 5 x)\\\\
=&\ \cos 5 x \dfrac{d}{d x}(5 x) \\\\
=&\ 5 \cos 5 x\\\\\\
\text{(b)}\quad & \dfrac{d}{d x}\left[\cos \left(7 x^{2}-2\right)\right]\\\\
=&\ -\sin \left(7 x^{2}-2\right) \dfrac{d}{d x}\left(7 x^{2}-2\right) \\\\
=&\ -14 x \sin \left(7 x^{2}-2\right)\\\\\\
\text{(c)}\quad & \dfrac{d}{d x}[\tan (6 x+7)] \\\\
=&\ \sec ^{2}(6 x+7) \dfrac{d}{d x}(6 x+7) \\\\
=&\ 6 \sec ^{2}(6 x+7)\\\\\\
\text{(d)}\quad & \dfrac{d}{d x}[5 \sec (3 x+1)] \\\\
=&\ 5 \sec (3 x+1) \tan (3 x+1) \dfrac{d}{d x}(3 x+1) \\\\
=&\ 15 \sec (3 x+1) \tan (3 x+1)\\\\\\
\text{(e)}\quad & \dfrac{d}{d x}[\sin (2 x+3)] \\\\
=&\ \cos (2 x+3) \dfrac{d}{d x}(2 x+3) \\\\
=&\ 2 \cos (2 x+3)\\\\\\
\text{(f)}\quad & \dfrac{d}{d x}\left[\cos \left(\dfrac{3}{x}\right)\right] \\\\
=&\ -\sin \left(\dfrac{3}{x}\right) \dfrac{d}{d x}\left(\dfrac{3}{x}\right) \\\\
=&\ \dfrac{3}{x^{2}} \sin \left(\dfrac{3}{x}\right)\\\\\\
\text{(g)}\quad &\dfrac{d}{d x}\left(x^{3} \cos 2 x\right) \\\\
=&\ x^{3} \dfrac{d}{d x}(\cos 2 x)+\cos 2 x \dfrac{d}{d x}\left(x^{3}\right) \\\\
=&\ -2 x^{3} \sin 2 x+3 x^{2} \cos 2 x\\\\\\
\text{(h)}\quad & \dfrac{d}{d x}(\cos 7 x+\sin 3 x) \\\\
=&\ -\sin 7 x \dfrac{d}{d x}(7 x)+\cos 3 x \dfrac{d}{d x}(3 x) \\\\
=&\ -7 \sin 7 x+3 \cos 3 x\\\\\\
\text{(i)}\quad & \dfrac{d}{d x}\left[\dfrac{\cot (1-2 x)}{3}\right] \\\\
=&\ -\dfrac{1}{3} \csc^{2}(1-2 x) \dfrac{d}{d x}(1-2 x) \\\\
=&\ \dfrac{2}{3} \csc^{2}(1-2 x)\\\\\\
\text{(j)}\quad & \dfrac{d}{d x}(-2 \csc 3 x) \\\\
=&\ -2(-\csc 3 x \cot 3 x) \dfrac{d}{d x}(3 x) \\\\
=&\ 6 \csc 3 x \cot 3 x\\\\\\
\text{(k)}\quad & \dfrac{d}{d x}(\sin x \cos 2 x)\\\\
=&\ \sin x \dfrac{d}{d x}(\cos 2 x)+\cos 2 x \dfrac{d}{d x}(\sin x)\\\\
=&\ -\sin x \sin 2 x \dfrac{d}{d x}(2 x)+\cos x \cos 2 x\\\\
=&\ -2 \sin x \sin 2 x+\cos x \cos 2 x\\\\\\
\text{(l)}\quad & \dfrac{d}{d x}\left[\cos ^{2}(5 x)\right]\\\\
=&\ 2 \cos 5 x \dfrac{d}{d x}(\cos 5 x)\\\\
=&\ -2 \sin 5 x \cos 5 x \dfrac{d}{d x}(5 x)\\\\
=&\ -10 \sin 5 x \cos 5 x\\\\\\
\text{(m)}\quad & \dfrac{d}{d x}\left(\tan ^{3} \sqrt{x}\right)\\\\
=&\ 3 \tan ^{2} \sqrt{x} \dfrac{d}{d x}(\tan \sqrt{x})\\\\
=&\ 3 \tan ^{2} \sqrt{x} \sec ^{2} \sqrt{x} \dfrac{d}{d x}(\sqrt{x})\\\\\\
\text{(n)}\quad & \dfrac{d}{d x}[\sin (\cos x)] \\\\
=& \cos (\cos x) \dfrac{d}{d x}(\cos x) \\\\
=&-\sin x[\cos (\cos x)] \\\\\\
\text{(o)}\quad & \dfrac{d}{d x}\left(\dfrac{\sin x}{1+\tan x}\right) \\\\
=& \dfrac{(1+\tan x) \dfrac{d}{d x}(\sin x)-\sin x \dfrac{d}{d x}(1+\tan x)}{(1+\tan x)^{2}} \\\\
=& \dfrac{\cos x(1+\tan x)-\sin x \cdot \sec ^{2} x}{(1+\tan x)^{2}}\\\\\\
\text{(p)}\quad & \dfrac{d}{d x} \sqrt{\sin x+\cos x} \\\\
=& \dfrac{1}{2 \sqrt{\sin x+\cos x}} \dfrac{d}{d x}(\sin x+\cos x) \\\\
=& \dfrac{\cos x-\sin x}{2 \sqrt{\sin x+\cos x}} \\\\\\
\text{(q)}\quad & \dfrac{d}{d x}(x+\tan x)^{3} \\\\
=& 3(x+\tan x)^{2} \dfrac{d}{d x}(x+\tan x) \\\\
=& 3(x+\tan x)^{2}\left(1+\sec ^{2} x\right) \\\\\\
\text{(r)}\quad & \dfrac{d}{d x}\left(\dfrac{\tan 2 x}{1+\cot x}\right) \\\\
=& \dfrac{(1+\cot x) \dfrac{d}{d x}(\tan 2 x)-(\tan 2 x) \dfrac{d}{d x}(1+\cot x)}{(1+\cot x)^{2}} \\\\
=& \dfrac{2 \tan 2 x(1+\cot x)+\tan 2 x \csc ^{2} x}{(1+\cot x)^{2}}
\end{aligned}$
SOLUTION
$\begin{aligned}
\text{(a)}\quad y &=\sin ^{2} x \\\\
\dfrac{d y}{d x} &=2 \sin x \dfrac{d}{d x}(\sin x) \\\\
&=2 \sin x \cos x \\\\
&=\sin 2 x \\\\\\
\text{(b)}\quad y &=\cos \sqrt{x} \\\\
\dfrac{d y}{d x} &=2(-\sin \sqrt{x}) \dfrac{d}{d x}(\sqrt{x}) \\\\
&=-\dfrac{\sin \sqrt{x}}{\sqrt{x}} \\\\\\
\text{(c)}\quad y &=\tan ^{2}\left(x^{2}\right) \\\\
\dfrac{d y}{d x} &=2 \tan \left(x^{2}\right) \dfrac{d}{d x}\left[\tan \left(x^{2}\right)\right] \\\\
&=2 \tan \left(x^{2}\right) \sec ^{2}\left(x^{2}\right) \dfrac{d}{d x}\left(x^{2}\right) \\\\
&=4 x \tan \left(x^{2}\right) \sec ^{2}\left(x^{2}\right)\\\\\\
\text{(d)}\quad y &=\sin 2 x-x \cos x \\\\
\dfrac{d y}{d x} &=\cos 2 x \dfrac{d}{d x}(2 x)-(-x \sin x+\cos x) \\\\
&=2 \cos 2 x+x \sin x-\cos x \\\\\\
\text{(e)}\quad y &=\dfrac{x}{\tan x} \\\\
\dfrac{d y}{d x} &=\dfrac{\tan x-x \sec ^{2} x}{\tan ^{2} x} \\\\
&=\dfrac{1}{\tan x}-x \dfrac{\cos ^{2} x}{\sin ^{2} x} \dfrac{1}{\cos ^{2} x} \\\\
&=\cot x-x \operatorname{cosec}^{2} x \\\\\\
\text{(f)}\quad y &=\sin x \cos ^{2} x \\\\
\dfrac{d y}{d x} &=\sin x(2 \cos x) \dfrac{d}{d x}(\cos x)+\cos ^{2} x \cdot \cos x \\\\
&=-2 \sin ^{2} x \cos x+\cos ^{3} x\\\\\\
\text{(g)}\quad y &=\sin \left(1-x^{2}\right) \\\\
\dfrac{d y}{d x} &=\cos \left(1-x^{2}\right) \dfrac{d}{d x}\left(1-x^{2}\right) \\\\
&=-2 x \cos \left(1-x^{2}\right) \\\\\\
\text{(h)}\quad y &=2 \pi x+2 \cos \pi x \\\\
\dfrac{d y}{d x} &=2 \pi-2 \sin \pi x \dfrac{d}{d x}(\pi x) \\\\
&=2 \pi(1-\sin \pi x) \\\\\\
\text{(i)}\quad y &=\sin ^{2} x \cos 3 x \\\\
\dfrac{d y}{d x} &=-\sin ^{2} x \sin 3 x \dfrac{d}{d x}(3 x)+\cos 3 x(2 \sin x) \dfrac{d}{d x}(\sin x) \\\\
&=-3 \sin ^{2} x \sin 3 x+2 \sin x \cos x \cos 3 x \\\\
&=-3 \sin ^{2} x \sin 3 x+\sin 2 x \cos 3 x \\\\\\
\text{(j)}\quad y &=x^{2} \sin \left(\dfrac{1}{x}\right) \\\\
\dfrac{d y}{d x} &=x^{2} \cos \left(\dfrac{1}{x}\right) \dfrac{d}{d x}\left(\dfrac{1}{x}\right)+2 x \sin \left(\dfrac{1}{x}\right) \\\\
&=x^{2}\left(-\dfrac{1}{x^{2}}\right) \cos \left(\dfrac{1}{x}\right)+2 x \sin \left(\dfrac{1}{x}\right) \\\\
&=-\cos \left(\dfrac{1}{x}\right)+2 x \sin \left(\dfrac{1}{x}\right)\\\\\\
\text{(k)}\quad & 3 x^{2}+2 \sin y=y^{2}\\\\
&\text{Differentiate with respect to } x,\\\\
&6 x+2 \cos y \dfrac{d y}{d x}=2 y \dfrac{d y}{d x} \\\\
&\dfrac{d y}{d x}=\dfrac{3 x}{y-\cos y} \\\\\\
\text{(l)}\quad & \sin x \cos y=2 y\\\\
&\text{Differentiate with respect to } x,\\\\
&-\sin x \sin y \dfrac{d y}{d x}+\cos x \cos y=2 \dfrac{d y}{d x} \\\\
&\dfrac{d y}{d x}=\dfrac{\cos x \cos y}{2+\sin x \sin y} \\\\\\
\text{(m)}\quad &2 x y+\sin x=3\\\\
&\text{Differentiate with respect to } x,\\\\
&2 x \dfrac{d y}{d x}+2 y+\cos x=0 \\\\
&\dfrac{d y}{d x}=-\dfrac{\cos x+2 y}{2 x}\\\\\\
\text{(n)}\quad y &=\dfrac{\cos (1-2 x)}{\sqrt{x}} \\\\
\dfrac{d y}{d x} &=\dfrac{-\sqrt{x} \sin (1-2 x) \dfrac{d}{d x}(1-2 x)-\dfrac{\cos (1-2 x)}{2 \sqrt{x}}}{x} \\\\
&=\dfrac{1}{x}\left[2 \sqrt{x} \sin (1-2 x)-\dfrac{\cos (1-2 x)}{2 \sqrt{x}}\right] \\\\
&=\dfrac{4 x \sin (1-2 x)-\cos (1-2 x)}{2 x \sqrt{x}} \\\\\\
\text{(o)}\quad y &=\sin ^{4} x \cos (3 x) \\\\
\dfrac{d y}{d x} &=-\sin ^{4} x \sin 3 x \dfrac{d}{d x}(3 x)+4 \sin ^{3} x \cos (3 x) \dfrac{d}{d x}(\sin x) \\\\
&=-3 \sin ^{4} x \sin 3 x+4 \sin ^{3} x \cos (3 x) \cos x \\\\\\
\text{(p)}\quad & x+ \sin y=\cos (x y) \\\\
&\text{Differentiate with respect to } x,\\\\
&1+\cos y \dfrac{d y}{d x}=-\sin (x y) \dfrac{d}{d x}(x y) \\\\
&1+\cos y \dfrac{d y}{d x}=-\sin (x y)\left(x \dfrac{d y}{d x}+y\right) \\\\
&{[x \sin (x y)+\cos y] \dfrac{d y}{d x}=-[1+y \sin (x y)]} \\\\
&\dfrac{d y}{d x}=-\dfrac{1+y \sin (x y)}{x \sin (x y)+\cos y} \\\\\\
\text{(q)}\quad & x+\sin y=\cos (x y)\\\\
&\text{Differentiate with respect to } x,\\\\
&1+\cos y \dfrac{d y}{d x}=-\sin (x y) \dfrac{d}{d x}(x y) \\\\
&1+\cos y \dfrac{d y}{d x}=-\sin (x y)\left(x \dfrac{d y}{d x}+y\right) \\\\
&{[x \sin (x y)+\cos y] \dfrac{d y}{d x}=-[1+y \sin (x y)]} \\\\
&\dfrac{d y}{d x}=-\dfrac{1+y \sin (x y)}{x \sin (x y)+\cos y} \\\\\\
\text{(r)}\quad & x+y^{2}=\sin (x+y)\\\\
&1+2 y \dfrac{d y}{d x}=\cos (x+y) \dfrac{d}{d x}(x+y) \\\\
&1+2 y \dfrac{d y}{d x}=\cos (x+y)\left(1+\dfrac{d y}{d x}\right) \\\\
&{[2 y-\cos (x+y)] \dfrac{d y}{d x}=\cos (x+y)-1} \\\\
&\dfrac{d y}{d x}=\dfrac{\cos (x+y)-1}{2 y-\cos (x+y)}
\end{aligned}$
SOLUTION
$\begin{aligned}
y &=x \sin x \\\\
\frac{d y}{d x} &=x \cos x+\sin x \\\\
\frac{d^{2} y}{d x^{2}} &=-x \sin x+\cos x+\cos x \\\\
&=2 \cos x-x \sin x
\end{aligned}$
SOLUTION
$\begin{aligned}
y &=\cos ^{2} x \\\\
\frac{d y}{d x} &=2 \cos x \frac{d}{d x}(\cos x) \\\\
&=-2 \sin x \cos x \\\\
&=-\sin 2 x \\\\
\frac{d^{2} y}{d x^{2}} &=-\cos 2 x \frac{d}{d x}(2 x) \\\\
&=-2 \cos 2 x \\\\
\frac{d^{2} y}{d x^{2}}+4 y &=-2 \cos 2 x+4 \cos ^{2} x \\\\
&=-2\left(\cos ^{2} x-\sin ^{2} x\right)+4 \cos ^{2} x \\\\
&=-2 \cos ^{2} x+2 \sin ^{2} x+4 \cos ^{2} x \\\\
&=2\left(\sin ^{2} x+\cos ^{2} x\right) \\\\
&=2
\end{aligned}$
SOLUTION
$\begin{aligned}
y&= \frac{1}{3} \cos ^{3} x-\cos x \\\\
\frac{d y}{d x} &=\cos ^{2} x \frac{d}{d x}(\cos x)-(-\sin x) \\\\
&=-\sin x \cos ^{2} x+\sin x \\\\
&=\sin x\left(1-\cos ^{2} x\right) \\\\
&=\sin x\left(\sin ^{2} x\right) \\\\
&=\sin ^{3} x
\end{aligned}$
SOLUTION
$\begin{aligned}
&x \cos y=\sin x\\\\
&\text{Differentiate with respect to } x,\\\\
&-x \sin y \frac{d y}{d x}+\cos y=\cos x \\\\
\therefore \quad & \frac{d y}{d x}=\frac{1}{x} \cdot \frac{\cos y-\cos x}{\sin y} \\\\
& \text { Since } x \cos y=\sin x, \frac{1}{x}=\frac{\cos y}{\sin x} \\\\
\therefore \quad & \frac{d y}{d x}=\frac{\cos y(\cos y-\cos x)}{\sin y \sin x}
\end{aligned}$


Posting Komentar untuk "Calculus Exercise (8) : Derivative of Trigonometric Functions"